
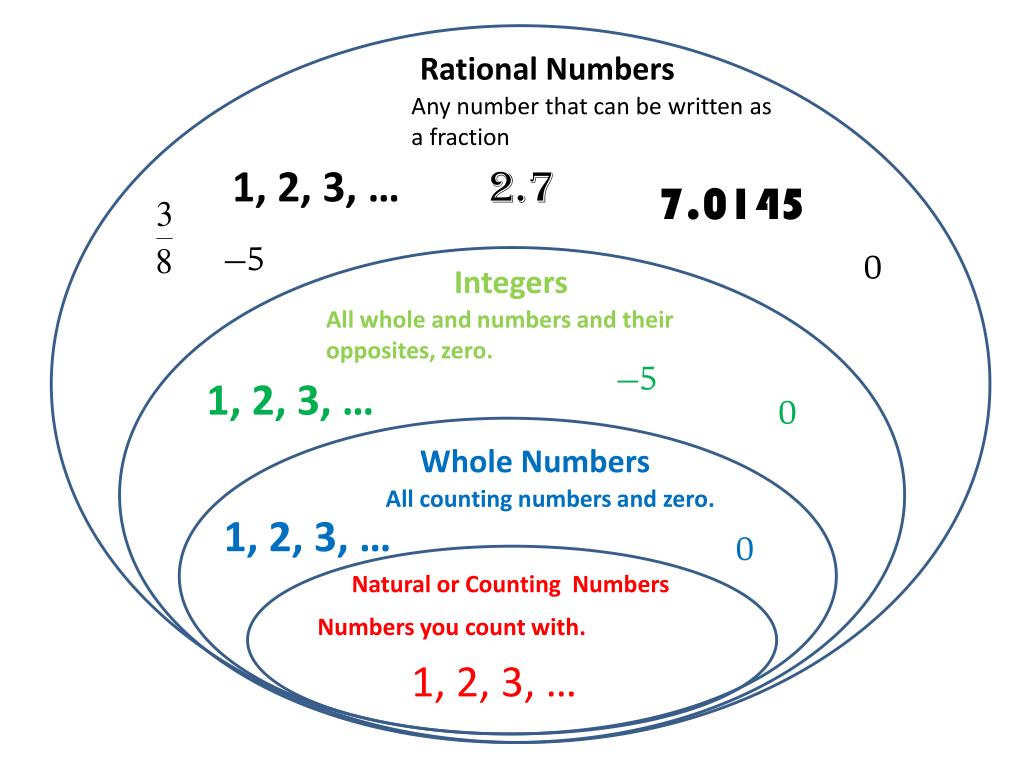
We need to look at all the numbers we have used so far and verify that they are rational. A few examples areĤ 5, − 7 8, 13 4, and − 20 3 4 5, − 7 8, 13 4, and − 20 3Įach numerator and each denominator is an integer. Īll fractions, both positive and negative, are rational numbers. Do you remember what the difference is among these types of numbers?Ī rational number is a number that can be written in the form p q, p q, where p p and q q are integers and q ≠ o. We have already described numbers as counting numbers, whole numbers, and integers.

And we'll practice using them in ways that we'll use when we solve equations and complete other procedures in algebra. We'll work with properties of numbers that will help you improve your number sense. We'll take another look at the kinds of numbers we have worked with in all previous chapters. In this chapter, we'll make sure your skills are firmly set. You have established a good solid foundation that you need so you can be successful in algebra. You have solved many different types of applications. You have become familiar with the language and symbols of algebra, and have simplified and evaluated algebraic expressions.
ALL INTEGERS ARE RATIONAL NUMBERS HOW TO
You have learned how to add, subtract, multiply, and divide whole numbers, fractions, integers, and decimals.
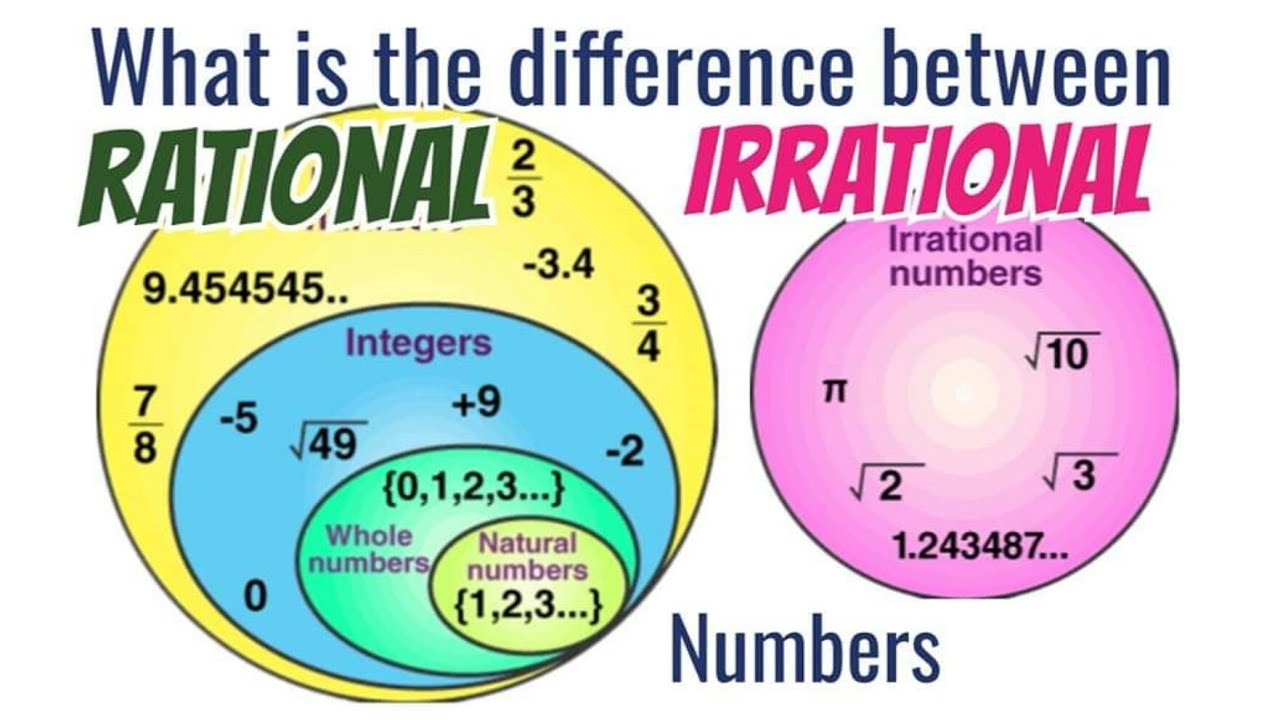
Identify Rational Numbers and Irrational NumbersĬongratulations! You have completed the first six chapters of this book! It's time to take stock of what you have done so far in this course and think about what is ahead. Definition Ī set S, maps to a different natural number, a difference between two n-tuples by a single element is enough to ensure the n-tuples being mapped to different natural numbers.\) referring to countable and countably infinite respectively, but as definitions vary the reader is once again advised to check the definition in use. The terms enumerable and denumerable may also be used, e.g. The reader is advised to check the definition in use when encountering the term "countable" in the literature. To avoid ambiguity, one may limit oneself to the terms "at most countable" and "countably infinite", although with respect to concision this is the worst of both worlds. An alternative style uses countable to mean what is here called countably infinite, and at most countable to mean what is here called countable.
The concept is attributed to Georg Cantor, who proved the existence of uncountable sets, that is, sets that are not countable for example the set of the real numbers.Īlthough the terms "countable" and "countably infinite" as defined here are quite common, the terminology is not universal. A countable set that is not finite is said to be countably infinite. In more technical terms, assuming the axiom of countable choice, a set is countable if its cardinality (the number of elements of the set) is not greater than that of the natural numbers. Equivalently, a set is countable if there exists an injective function from it into the natural numbers this means that each element in the set may be associated to a unique natural number, or that the elements of the set can be counted one at a time, although the counting may never finish due to an infinite number of elements. In mathematics, a set is countable if either it is finite or it can be made in one to one correspondence with the set of natural numbers. Not to be confused with (recursively) enumerable sets.
For the statistical concept, see Count data. For the linguistic concept, see Count noun.


 0 kommentar(er)
0 kommentar(er)
